Answer:
Equation A is non-linear.
Explanation:
We are given four equations in which we need to determine if they are linear or non-linear relationships.
Firstly, we need to know some information about linear equations:
- Linear equations cannot have an exponent.
- Linear equations have a constant slope and are straight lines.
- Linear equations can be negative or positive.
- Linear equations have a domain of all real numbers. They also have a range of all real numbers.
Now, in order to check this easily, we need to place these equations into slope-intercept form.
Equation A
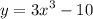
We see that this has an exponent, so this cannot be a linear equation.
In fact, because it is cubed, this is called a cubic function. Therefore, equation A is not a linear equation.
Equation B
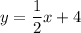
This equation does not have a exponent. It also has a slope and a y-intercept. Therefore, equation B is a linear equation.
Equation C
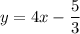
The equation does not have a exponent. It also has a slope and a y-intercept. Therefore, equation C is a linear equation.
Equation D
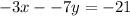
First off, we need to get this equation into slope-intercept form, if possible.

Our final equation is
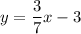 .
.
The equation does not have an exponent. It has a constant slope and a y-intercept. Therefore, equation D is a linear equation.
Let's check our equations:
- Equation A:
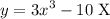
- Equation B:
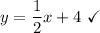
- Equation C:
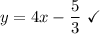
- Equation D:
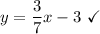
Therefore, we have determined that Equation A is not a linear equation, making it non-linear.