Question

Consider the series 1/4 1/16 1/64 1/256 which expression defines sn

Answer 1

This is a geometric progression

• Common ratio=1/16÷1/4 =1/4
• first term =a=1/4

So

The general formUla is

`\\ \rm\Rrightarrow a(n)=ar^(n-1)`

`\\ \rm\Rrightarrow a(n)=(1)/(4)\left((1)/(4)\right)^(n-1)`

Answer 2

`\displaystyle \lim_(n \to \infty) (1)/(3)\left(1-\left((1)/(4)\right)^n\right)`

Explanation:

Series: the sum of the elements of a sequence.

Therefore, as the numbers have been defined as a series and we need to find
`S_n`:

`(1)/(4)+(1)/(16)+(1)/(64)+(1)/(256)+...`

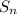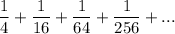
First determine if the sequence is arithmetic or geometric.

If it is an arithmetic sequence, there will be a common difference between consecutive terms.

if it is a geometric sequence, there will be a common ratio between consecutive terms.

From inspection of the terms, we can see that there is a common ratio of 1/4, as each term is the previous term multiplied by 1/4, so it is a geometric series.

Sum of the first n terms of a geometric series:

`S_n=(a(1-r^n))/(1-r)`

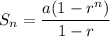
Given:

`a=(1)/(4)`

`r=(1)/(4)`

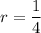
Substitute the values of a and r into the formula:

`\implies S_n=((1)/(4)\left(1-\left((1)/(4)\right)^n\right))/(1-(1)/(4))`

`\implies S_n=((1)/(4)\left(1-\left((1)/(4)\right)^n\right))/((3)/(4))`

`\implies S_n=(1)/(3)\left(1-\left((1)/(4)\right)^n\right)`

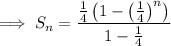
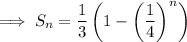
Therefore:

`\displaystyle \lim_(n \to \infty) (1)/(3)\left(1-\left((1)/(4)\right)^n\right)`