Answer:
Solution : Option B, or 9π
Explanation:
We are given that y = x, x = 3, and y = 0.
Now assume we have a circle that models the given information. The radius will be x, so to determine the area of that circle we have πx². And knowing that x = 3 and y = 0, we have the following integral:
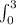
So our set up for solving this problem, would be such:

By solving this integral we receive our solution:
![\int _0^3x^2\pi dx,\\\mathrm{Take\:the\:constant\:out}:\quad \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx\\=> \pi \cdot \int _0^3x^2dx\\\mathrm{Apply\:the\:Power\:Rule}:\quad \int x^adx=(x^(a+1))/(a+1)\\=> \pi \left[(x^(2+1))/(2+1)\right]^3_0\\=> \pi \left[(x^3)/(3)\right]^3_0\\\mathrm{Compute\:the\:boundaries}: \left[(x^3)/(3)\right]^3_0=9\\\mathrm{Substitute:9\pi }](https://img.qammunity.org/2021/formulas/mathematics/college/2auyitzabg7xcw1ujr7zz9xt7llt440unx.png)
As you can tell our solution is option b, 9π. Hope that helps!