Answer:
4092
Explanation:
The n th term of a geometric sequence is
 = a
= a
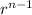
where a is the first term and r the common ratio
4
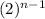 ← is the n th term of a geometric sequence
← is the n th term of a geometric sequence
with a = 4 and r = 2
The sum to n terms of a geometric sequence is
 =
=
 , thus
, thus
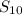 =
=
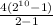 = 4(1024 - 1) = 4 × 1023 = 4092
= 4(1024 - 1) = 4 × 1023 = 4092