Answer:
The geometric mean of the sequence is 1814.99.
Explanation:
The formula to compute the geometric mean is:
![GM=\sqrt[n]{a_(1)\cdot a_(2)\cdot a_(3)\cdot...a_(n)}](https://img.qammunity.org/2021/formulas/mathematics/college/8r6fa70u1a3wl5z0xu7uw5gvdswu3lroqg.png)
The geometric sequence is:
–14, ? , ? , ? , ? , –235,298
The nth term of a geometric sequence is:

Here, r is the common ratio.
There are 6 terms in the sequence provided.
Compute the common ratio as follows:
![a_(6)=a_(1)\cdot r^(6-1)\\\\-235298=-14* r^(5)\\\\r=[(235298)/(14)]^(1/5)\\\\r=7](https://img.qammunity.org/2021/formulas/mathematics/college/ubk7eg49g2nsqr9bcuye3e0egzfbzevrhc.png)
Thus, the common ratio is 7.
The missing terms are:
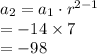
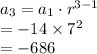
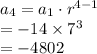

Compute the geometric mean as follows:
![GM=\sqrt[n]{a_(1)\cdot a_(2)\cdot a_(3)\cdot...a_(n)}](https://img.qammunity.org/2021/formulas/mathematics/college/8r6fa70u1a3wl5z0xu7uw5gvdswu3lroqg.png)
![=\sqrt[6]{-14* -98* -686* -4802*-33614* -235298} \\\\=1814.9854\\\\\approx 1814.99](https://img.qammunity.org/2021/formulas/mathematics/college/tf4kph8i8dx93kyxv1afeo5p1bfbskeitw.png)
Thus, the geometric mean of the sequence is 1814.99.