Question

The lateral area of a right circular cone is equal to 120 pi cm^2. if the base of the cone has a diameter of 24 cm , what is the length of the slant height

Answer 1

Slant height is equal to 15.62 cm

Explanation:

Diameter= 24cm

Radius = 12cm

Area of cone is = πrl

120πcm² = πrl

rl = 120

12l = 120

l = 120/12

l =10

If the height is 10 and radius is 12..

Using right angle triangle method, let the slant height be x

X² = 10² + 12²

X² = 100 + 144

X² = 244

X = √244

X= 15.62

Slant height is equal to 15.62 cm

Answer 2

10cm

Explanation:

Given a cone of base radius r and slant height l, the Lateral Area (Curved Surface Area) of the cone is determined using the formula:

Lateral Area of a Cone =
`\pi rl`

Diameter of the Cone = 24cm

Radius = Diameter/2 =24/2 =12 cm

Therefore:

Lateral Area of a Cone =
`\pi rl`

`120 \pi=12 \pi l\\l=10`

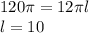
Therefore, the slant height of the cone is 10cm