Question

Find dy/dx for y= 4^(lnx)

Answer 1

dy/dx = (4^lnx)(ln4)/x

Explanation:

lny = (lnx)ln4

(1/y)y' = (ln4)/x

y' = y(ln4)/x

y' = (4^lnx)(ln4)/x

Answer 2

`y'=\ln(4)(4^(\ln(x)))/(x)`

Explanation:

`y=4^(\ln(x))`

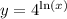
Take natural log of both sides:

`\ln(y)=\ln(4^(\ln(x)))`

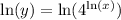
Use power rule of logarithms:

`\ln(y)=\ln(x)\cdot\ln(4)`

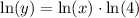
Let's differentiate.

`(y')/(y)=(1)/(x) \cdot \ln(4)`

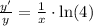
I applied chain rule on the left side and I apply constant multiple rule to the right side.

Let's multiply both sides by
`y`:

`y'=y \cdot (1)/(x) \cdot \ln(4)`

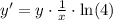
We started with
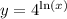`y=4^(\ln(x))` so let's make that replacement:

`y'=4^(\ln(x)) \cdot (1)/(x) \cdot \ln(4)`

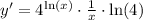
Let's simplify it a bit:

`y'=\ln(4)(4^(\ln(x)))/(x)`