Given:
The quadratic function is:
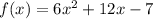
To find:
The zeros of the quadratic function.
Solution:
Quadratic formula: If a quadratic equation is
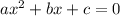 , then zeros of the quadratic equation are:
, then zeros of the quadratic equation are:

We have,
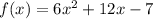
For zeros,
 .
.
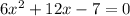
Here,
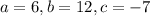 . Using quadratic formula, we get
. Using quadratic formula, we get
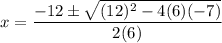
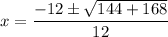
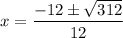
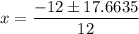
Now,
 and
and

 and
and
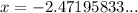
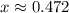 and
and
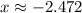
Therefore, the zeros of the given quadratic function are 0.472 and -2.472.