Question

Rewrite the equation by completing the square. 4 x^{2} +8 x +3 = 0

Answer 1

Answer:
(x+1)^2 = 1/4
Explanation:
4x ^ 2 + 8x + 3 = 0
Subtract 3 from each side
4x ^ 2 + 8x + 3-3 = 0-3
4x ^ 2 + 8x = -3
Divide each side by 4
x^2 +2x = -3/4
Take the coefficient of x
2
divide by 2
2/2 =1
Then square it
1^2 =1
Add this to each side
x^2 +2x = -3/4
x^2+2x+1 = -3/4+1
(x+1)^2 = 1/4

Answer 2

`\implies \boxed{\red{\sf ( x +1)^2 = (1)/(4)}}`

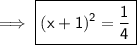
Explanation:

Given :-

• A equation is given to us
• The equation is 4x² + 8x + 3 = 0

And we need to write the equation by completing the square. Here's the step by step explanation .

Step 1: Make the coefficient of x² as 1 :-

`\implies (4x^2)/(4) + (8x)/(4) + (3 )/(4)= 0`

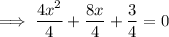
Step 2: Rewrite the equation :-

`\implies x^2 + 2x +(3)/(4)= 0`

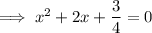
Step 3: Add 1² to both sides :-

`\implies x^2 + 2x + 1^2+(3)/(4)= 0 + 1^2`

Step 4: Rewriting in whole square form:-

`\implies ( x +1)^2 = 1 - (3)/(4)`

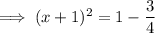
Step 5: Simplify the RHS :-

`\implies ( x +1)^2 = (4- 3)/(4)`

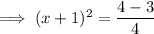
Step 6: The required form of equⁿ :-

`\implies ( x +1)^2 = (1)/(4)`

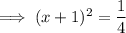
Hence the equation by rewriting it by completing the square is ( x + 1)² = 1/4 .