Answer:
The height is 14.86 yards ⇒ answer C
Explanation:
* Lets explain how to solve the problem
- The flower garden in the shape of a trapezoid
- The shorter base to be 3 yards greater than the height
- The longer base to be 7 yards greater than the height
- The area must be 295 square yards
- The situation is modeled by the equation h² + 5h = 295
- We want to find the height that will give the desired area by using
the quadratic formula
- The quadratic formula is
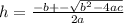 ,
,
where a is the coefficient of h² and b is the coefficient of h and c
is the numerical term
- The equation of the area is h² + 5h = 295
∵ h² + 5h = 295
- Subtract 295 from both sides
∴ h² + 5h - 295 = 0
- Lets find the values of a , b and c from the equation
∵ a = 1 , b = 5 , c = -295
∴
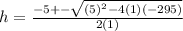
∴
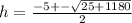
∴
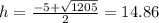
- OR
∴
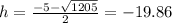
- The dimensions of any figure must be positive value, then we will
neglect the negative value of h
∴ h = 14.86
* The height is 14.86 yards