Question

Let f(x)= sqrt 6x and g(x)=x+3 smallest number that is in the domain of

Answer 1

Answer: -3 is the answer.

Step-by-step explanation:

Answer 2

***The complete question is: Let f(x)= sqrt 6x and g(x)=x+3 smallest number that is in the domain of f(g(x))***

Answer: The smallest number that is in the domain is -3.

Step-by-step explanation:

Given functions:

`f(x) = √(6x)`

`g(x) = x + 3`

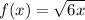
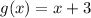
To find f(g(x)), put g(x) in f(x) as follows:

`f(g(x)) = f(x+3) = √(6(x+3)) = √(6x + 18)`

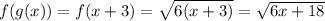
As (6x+18) is in square-root (and square-root of a negative number is an imaginary value), therefore, the domain should be the following:

`6x + 18 \geq 0\\6x \geq -18\\x \geq -3`

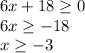
Therefore, the domain is [-3, +∞).

So the smallest number that is in the domain is -3.