Answer:
The solution of the equation is d=1.
Explanation:
Given : Equation
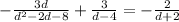
To find : What is the solution to the equation?
Solution :
Step 1 - Write the equation
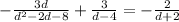
Step 2 - Factor the quadratic equation and take RHS to LHS
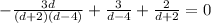
Step 3 - Take the Least common denominator (LCD)
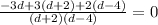
Step 4 - Solve the numerator and take denominator to other side
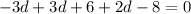
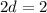
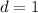
Therefore, The solution of the equation is d=1.