Question

If the zeros of a quadratic function F are 1 and 5 What is the equation of the axis of symmetry of f

Answer 1

it is x=3

you’re welcome

Answer 2

Option 2 is correct.

The axis of symmetry is, x= 3

Explanation:

Given: The zeros of the quadratic function f are 1 and 5.

If the zeroes are at x =1 and at x =5, then,

the factor equations were x- 1=0 and x-5 = 0.

Then. the factors were x -1 and x-5 .

Any factorable quadratic is going to have just the two factors, so these are must be them.

Then, the original quadratic was :

`(x-1)(x-5)= 0`

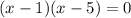
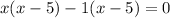`x(x-5) -1(x-5) =0` or

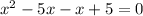`x^2-5x -x+5 =0` [by distributive property
`a\cdot (b+c) =a\cdot b+a\cdot c` ]

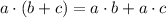Combine like terms;

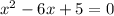`x^2-6x+5 =0` ......[1]

A quadratic equation is of the form:
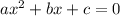`ax^2+bx+c =0`; where a, b, c are the coefficient ]

On comparing equation [1] with general equation we have;

the value of a = 1 , b = -6 and c =5

Axis of symmetry states that a parabola is a vertical line that divides the parabola into two congruent halves.

i,e
`x =-(b)/(2a)`

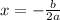
Then;

Axis of symmetry (x) =
`-(-6)/(2 \cdot 1) = (6)/(2) = 3`

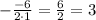
Therefore, the equation of the axis of symmetry is; x = 3