reletive extrema are where the first derivitive is 0 and the sign of the first derivitive changes
inflection points are where the 2nd derivitive is 0 and the sign of the 2nd derivitive changesd
I use f'(x)=1st derivitive and f''(x)=2nd derivitive
remember power rule:
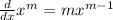
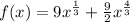
f'(x)
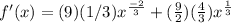
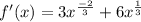
f''(x)
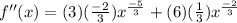
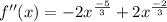
I'm not going to show the work for solving for when f'(x)=0 and f''(x)=0 because at this stage of the game, you should be able to do that easily
f'(x) is equal to 0 when x=-0.5, the sign changes from negative to positive at this point so at this point, the function has a relative minimum
f''(x) is equal to 0 when x=1, the sign changes from negative to positive at this point so at this point, the function is changing from concave down to concave up