Question

If A^2=A, which matrix is matrix A

Answer 1

Options 1 and 3.

Explanation:

By definition the product between two matrices is:

Let's suppose both matrices are 2x2,

`A=\left[\begin{array}{cc}a_(11)&a_(12)\\a_(21)&a_(22)\end{array}\right]`

`B=\left[\begin{array}{cc}b_(11)&b_(12)\\b_(21)&b_(22)\end{array}\right]`

The product between A and B is:

`AB=\left[\begin{array}{cc}a_(11)b_(11)+a_(12)b_(21)&a_(11)b_(12)+a_(12)b_(22)\\a_(21)b_(11)+a_(22)b_(21)&a_(21)b_(12)+a_(22)b_(22)\end{array}\right]`

IMPORTANT: It's not the same AB then BA the results of both products are differents.

Now we are going to analyze every option:

`A^2=A.A`

Option 1:

`A=\left[\begin{array}{cc}5&5\\-4&-4\end{array}\right]`

`A.A=\left[\begin{array}{cc}5&5\\-4&-4\end{array}\right] .\left[\begin{array}{cc}5&5\\-4&-4\end{array}\right] =\\\\=\left[\begin{array}{cc}5.5+5(-4)&5.5+5(-4)\\(-4).5+(-4).(-4)&(-4).5+(-4)(-4)\end{array}\right] \\\\=\left[\begin{array}{cc}25-20&25-20\\-20+16&-20+16\end{array}\right] \\\\=\left[\begin{array}{cc}5&5\\-4&-4\end{array}\right]=A`

We can see that A.A=A then this is the correct option.

Option 2:

`A=\left[\begin{array}{cc}6&5\\5&6\end{array}\right]\\\\A.A=\left[\begin{array}{cc}6&5\\5&6\end{array}\right].\left[\begin{array}{cc}6&5\\5&6\end{array}\right]\\\\=\left[\begin{array}{cc}6.6+5.5&6.5+5.6\\5.6+6.5&5.5+6.6\end{array}\right]\\\\=\left[\begin{array}{cc}36+25&30+30\\30+30&25+36\end{array}\right]\\\\=\left[\begin{array}{cc}61&60\\60&61\end{array}\right]\\eq A`

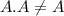`A.A\\eq A` Then this option is incorrect.

Option 3:

`A=\left[\begin{array}{cc}0,5&-0,5\\-0,5&0,5\end{array}\right] \\\\A.A=\left[\begin{array}{cc}0,5&-0,5\\-0,5&0,5\end{array}\right].\left[\begin{array}{cc}0,5&-0,5\\-0,5&0,5\end{array}\right]\\\\=\left[\begin{array}{cc}0,5.0,5+(-0,5).(-0,5)&0,5.(-0,5)+(-0,5).(0,5)\\(-0,5).0,5+0,5.(-0,5)&(-0,5).(-0,5)+0,5.0,5\end{array}\right]\\\\=\left[\begin{array}{cc}0,25+0,25&-0,25-0,25\\-0,25-0,25&0,25+0,25\end{array}\right]\\\\=\left[\begin{array}{cc}0,5&-0,5\\-0,5&0,5\end{array}\right]= A`

We can see that this option is also correct.

Option 4:

`A=\left[\begin{array}{cc}0,5&0,5\\-0,5&0,5\end{array}\right] \\\\A.A=\left[\begin{array}{cc}0,5&0,5\\-0,5&0,5\end{array}\right].\left[\begin{array}{cc}0,5&0,5\\-0,5&0,5\end{array}\right]\\\\=\left[\begin{array}{cc}0,5.0,5+0,5.(-0,5)&0,5.0,5+0,5.0,5\\(-0,5).0,5+0,5.(-0,5)&(-0,5).0,5+0,5.0,5\end{array}\right]\\\\=\left[\begin{array}{cc}0,25-0,25&0,25+0,25\\-0,25-0,25&-0,25+0,25\end{array}\right]\\\\=\left[\begin{array}{cc}0&0,5\\-0,5&0\end{array}\right]\\eq A`

Then this option is incorrect.

Option 5:

`A=\left[\begin{array}{cc}-6&-6\\5&5\end{array}\right]\\\\A.A=\left[\begin{array}{cc}-6&-6\\5&5\end{array}\right].\left[\begin{array}{cc}-6&-6\\5&5\end{array}\right]\\\\=\left[\begin{array}{cc}(-6).(-6)+(-6).5&(-6).(-6)+(-6).5\\5.(-6)+5.5&5.(-6)+5.5\end{array}\right]\\\\=\left[\begin{array}{cc}36-30&36-30\\-30+25&-30+25\end{array}\right]\\\\=\left[\begin{array}{cc}6&6\\-5&-5\end{array}\right]\\eq A`

Then this option is incorrect.

Answer 2

Consider all options:

A.

`\left[\begin{array}{cc}5&5\\-4&-4\end{array}\right] \cdot \left[\begin{array}{cc}5&5\\-4&-4\end{array}\right] =\left[\begin{array}{cc}5\cdot 5+5\cdot (-4)&5\cdot 5+5\cdot (-4)\\-4\cdot 5+(-4)\cdot (-4)&-4\cdot 5+(-4)\cdot (-4)\end{array}\right]=`

`=\left[\begin{array}{cc}5&5\\-4&-4\end{array}\right].`

This option is true.

B.

`\left[\begin{array}{cc}6&5\\5&6\end{array}\right] \cdot \left[\begin{array}{cc}6&5\\5&6\end{array}\right] =\left[\begin{array}{cc}6\cdot 6+5\cdot 5&6\cdot 5+5\cdot 6\\5\cdot 6+6\cdot 5&5\cdot 5+6\cdot 6\end{array}\right]=`

`=\left[\begin{array}{cc}61&60\\60&61\end{array}\right].`

This option is false.

C.

`=\left[\begin{array}{cc}0.5&-0.5\\-0.5&0.5\end{array}\right].`

This option is true.

D.

`=\left[\begin{array}{cc}0&0.5\\-0.5&0\end{array}\right].`

This option is false.

E.

`\left[\begin{array}{cc}-6&-6\\5&5\end{array}\right] \cdot \left[\begin{array}{cc}-6&-6\\5&5\end{array}\right] =\left[\begin{array}{cc}-6\cdot (-6)+(-6)\cdot 5&-6\cdot (-6)+(-6)\cdot 5\\5\cdot (-6)+5\cdot 5&5\cdot (-6)+5\cdot 5\end{array}\right]=`

`=\left[\begin{array}{cc}6&6\\-5&-5\end{array}\right].`

This option is false.

Answer: correct options are A and C.