We have the following equation:
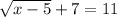
To solve this, we first need to subtract the 7 on both sides of the equation.
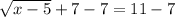
The equation then becomes:

Now we must square both sides of the equation to get rid of the radical/square root symbol.
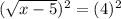
Now we have a normal one step equation.
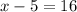
Add 5 on both sides to find x.
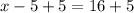
x=21
To see if this solution is true, we must substitute 21 back into the original equation.
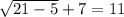
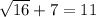
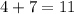
11=11
Therefore, the solution is true.