Question

at a particular school with 200 male students, 58 play football, 40 play basketball, and 8 play both. what is the probability that a randomly selected male student plays neither sport?

Answer 1

this helps (no play) = 121/200 = 0.6050

ifti doesn't I tried

Answer 2

`(11)/(20)`

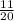
Explanation:

Total male students = 200

Students who play football (F)= 58

Students who play basketball (B)= 40

Students who play both (F∩B)= 8

The number of students who play either sport is

`(F\cup B)=F+B-(F\cap B)`

`(F\cup B)=58+40-8=90`

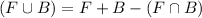
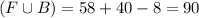
The number of students who play neither sport is

`200-(F\cup B)=200-90=110`

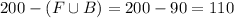
The probability that a randomly selected male student plays neither sport is

`Probability=\frac{\text{Number of students who play neither sport}}{\text{Total male students}}`

`Probability=(110)/(200)`

`Probability=(11)/(20)`

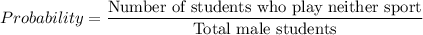
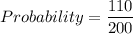
Hence, the required probability is
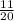`(11)/(20)`.