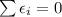Question

According to (1.17), lei = 0 when regression model (1.1) is fitted to a set of n cases by the method of least squares. is it also true that l e:i = o? comment.

Answer 1

Properties of Fitted Regression Line

Explanation:

We know that,

`\sum{e_(i) } = 0`

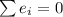
In turn we understand that

`e_(i)= Y_(i)-Y'_(i)`

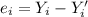
The third property of Fitted Regression Line tells us that: The sum of the observed values
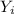`Y_(i)` equals the sum of the fitted values
`Y'_(i)`, so:

`\sum{Y_(i)} = \sum{Y'_(i)}` (1)

We further understand that the values given for
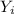`Y_(i)`, is equivalent to:

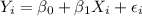`Y_(i)= \beta_(0) + \beta_(1)X_(i)+\epsilon_(i)` (2)

On the other hand for the definition of the value for the regression function of
`Y'_(i)` is,

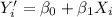`Y'_(i)= \beta_(0)+\beta_(1)X_(i)` (3)

By replacing (3) and (2) in (1), we get that

`\sum{ (\beta_(0) + \beta_(1)X_(i)+\epsilon_(i))} = \sum{(\beta_(0)+\beta_(1)X_(i))}`

Since the sum is distributive

`\sum \beta_(0) + \sum \beta_(1)X_(i)+\sum \epsilon_(i) = \sum\beta_(0)+ \sum \beta_(1)X_(i)`

Equal values on opposite sides of an equation are canceled, we get that

`\sum \epsilon_(i) = 0`

Answer 2

\sum ei =0

that is

\sum (yi-yiˆ)=0

means,

\sumyi = \sum yiˆ

yi = \alpha +\beta xi+ei

yiˆ=\alphaˆ+\betaˆxi

so,

\sumei=0

hence proved