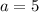Question

A parallelogram has side lengths of 4 and 6 and an angle of measure 55°.



Law of cosines: a2 = b2 + c2 – 2bccos(A)

What is x, the length of the diagonal, to the nearest whole number?

3

5

6

7

Answer 1

Answer: B

Explanation:

got it right on edge 2022

Answer 2

The Law of Cosines is a beautiful formula and a gateway to all sorts of wonders.

This question asking for the length to the nearest whole number is pretty ugly.

A diagonal of a parallelogram makes two congruent triangles. In this problem we're almost told we're interested in the diagonal opposite an A=55 degree angle, included between sides b=4 and c=6.


`a^2 = b^2 + c^2 - 2 b c \cos A`

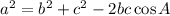
We just plug in the numbers.


`a^2 = 4^2 + 6^2 - 2(4)(6) \cos 55^\circ`


`a^2 = 52 - 48 \cos 55^\circ`

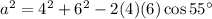
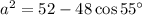
That's the exact answer, its square anyway. Now we approximate.


`\cos 55^\circ \approx .57`


`a^2 = 52 - 48(.57) = 24.6`

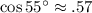
Taking the square root to the nearest integer,


`a = 5`