Question

The Fisher family bought a house for $191,000. They paid $40,000 down and took out a 15 year mortgage for the remaining balance at 5.25%, compounded monthly.

(a) What is their monthly payment?
(b) How much interest did they pay over the entire loan?

Answer 1

a) PMT=$1,213.86 ; b) Total interest = $67,494.80

Explanation:

a) In order to find their monthly payment we must start by determining the amount to be borrowed.

$191,000-$40,000=$151,000

Once we got this amount, we can make use of the annuity formula to fin the monthly payment:

`PMT=((r)/(n)(PV))/(1-(1+(r)/(n))^(-nt))`

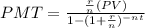
Where

PMT = monthly payment.

r = interest rate written as a decimal number.

n = number of times the interest will be compounded in a year.

PV = present value of the loan.

t = number of years.

So we know the following data:

r = 0.0525

n = 12

PV = $151,000

t = 15

So we can plug those numbers into the formula, so we get:

`PMT=((0.0525)/(12)(151,000))/(1-(1+(0.0525)/(12))^(-(12)(15)))`

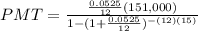
which solves to:

PMT=$1,213.86

b)

In order to find the interest they paid over the entire loan we must first find how much they paid in total for the loan. So we get the monthly payment and multiply that by the total number of months so we get:

Total=$1,213.86*12*15=$218,494.80

next, we subtract the original amount of the loan from that to determine how much of it is interest:

Total interest = $218,494.80-$151,000=$67,494.80

Answer 2

The monthly payment for loan paid by Fisher family is $1213.86 approx. The interest they paid over the entire loan is $67494.8

How to find the monthly payment for loan?

Let we have following figures:

• 
  `P_v =` present value of the loan
• r = rate of interest compounding per month
• t= time of loan payment
• n = number of times rate is compounded monthly,
• PMT = monthly loan payment to be done,

Then, we get this relation:

`PMT = ((r)/(n)* P_v)/(2 - (1 +(r)/(n) )^(-nt))`

The loan payment is total payment - down payment, which evaluates to:

`P_v = 191000 - 40000 = 151000`

Rate of interest = r = 5.25% = 0.0525 compounding monthly

Time for loan payment = t = 15 years

n = 12 (since compounding is done monthly here and there are 12 months in each year).

Thus, the monthly payment of loan for Fisher family is calculated as:

`PMT = ((r)/(n)* P_v)/(1 - (1 +(r)/(n) )^(-nt)) \\\\\\PMT = ((0.0525)/(12) * 151000)/(1 - (1+ (0.0525)/(12))^(-12 * 15) ) \approx 1213.86`(in dollars)

The total payment they made is this monthly payments times count of months in 15 years =
`1213.86 * 12 * 15 = 218494.8` (in dollars)

Thus, as the extra amount they paid is the amount of interest, therefore,

Interest they paid = Total amount paid - Original amount of loan

Interest they paid =
`218494.8 - 151000 = 67494.8` (in dollars).

Thus, the monthly payment for loan paid by Fisher family is $1213.86 approx. The interest they paid over the entire loan is $67494.8

Learn more about PMT here: