Question

A different species of cockroach has weights that are approximately Normally distributed with a mean of 50 grams. After measuring the weights of many of these cockroaches, a lab assistant reports that 14% of the cockroaches weigh more than 55 grams. Based on this report, what is the approximate standard deviation of weight for this species of cockroaches?

Answer 1

a 4.6 on the text BTW

Explanation:

bye good luck

Answer 2

The standard deviation of weight for this species of cockroaches is 4.62.

Explanation:

Given : A different species of cockroach has weights that are approximately Normally distributed with a mean of 50 grams. After measuring the weights of many of these cockroaches, a lab assistant reports that 14% of the cockroaches weigh more than 55 grams.

To find : What is the approximate standard deviation of weight for this species of cockroaches?

Solution :

We have given,

Mean
`\mu=50`

The sample mean x=55

A lab assistant reports that 14% of the cockroaches weigh more than 55 grams.

i.e. P(X>55)=14%=0.14

The total probability needs to sum up to 1,

`P(X\leq 55)=1-P(X>55)`

`P(X\leq 55)=1-0.14`

`P(X\leq 55)=0.86`

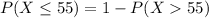
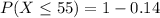
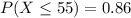
The z-score value of 0.86 using z-score table is z=1.08.

Applying z-score formula,

`z=(x-\mu)/(\sigma)`

Where,
`\sigma` is standard deviation

Substitute the values,

`z=(x-\mu)/(\sigma)`

`1.08=(55-50)/(\sigma)`

`1.08=(5)/(\sigma)`

`\sigma=(5)/(1.08)`

`\sigma=4.62`

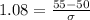
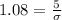
The standard deviation of weight for this species of cockroaches is 4.62.