Answer:
The total height and width of the poster is approximately 28.93 and 38.57.
Explanation:
Consider the provided information.
Let us consider the width and length of the printed part of the poster is x and y respectively.
For better understanding refer the figure 1:
The area of the printed part is 382 cm²
Area = xy = 382
y = 382/x
Now the total height of the poster is y + 6 + 6 = y + 12
Total width of the poster is x + 8 + 8 = x + 16
Thus the total area of the poster is:
A = (x + 16)(y + 12)
Now substitute the value of y in above equation.

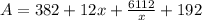

Now differentiate the above equation with respect to x.
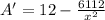
Now, substitute A'= 0 and solve for x.
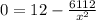
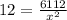
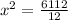
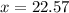 Ignore the negative value of x as width can't be a negative number.
Ignore the negative value of x as width can't be a negative number.
Now find A''
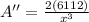
Here A" is positive for x>0 and x = 22.57 is minimum.
Use the value of x to find all the respective dimensions of the poster.
Substitute the value of x in y = 382/x
y = 382/22.57 = 16.93
The total height of the poster is y + 6 + 6 = y + 12 = 16.93 + 12 = 28.93
Total width of the poster is x + 8 + 8 = x + 16 = 22.57 + 16 = 38.57
Hence, the total height and width of the poster is approximately 28.93 and 38.57.