Question

HELP PLEASEE !!!
What is the left-hand limit of f(x)=|x-3|/x-3 as approaches 3?

Answer 1

-1

Explanation:

x-3 is negative when x aproaches 3 from the left so the limit can be rewritten as

`\lim_(x \to 3-) (-x+3)/(x-3)\\ = \lim_(x \to 3-) (-(x-3))/(x-3)\\\\=-1`

Answer 2

Option D -1

Step-by-step explanation:

Given : Function
`f(x)=(|x-3|)/(x-3)`

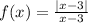
To find : What is the left-hand limit of function as approaches 3?

Solution :

Function
`f(x)=(|x-3|)/(x-3)`

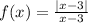
In left-hand limit,
`x\rightarrow 3^-`

`x<3\Rightarrow (x-3)<0\Rightarrow |x-3|=-(x-3)`

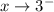
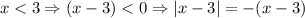
So,
`f(x)=(|x-3|)/(x-3)=(-(x-3))/(x-3)=-1,x\\eq3`

`\lim_(x\rightarrow3^-)f(x)= \lim_(x\rightarrow3^-) (-1)=-1`

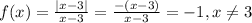
Therefore, The left-hand limit of function as approaches 3 is -1.

So, Option D is correct.