Question

Use the figure above to identify a pair of similar triangles, then find the scale factor. The image is not drawn to scale.

A. HEF ~EGH with a scale factor of root 3.2.
B. HEG-GEF with a scale factor of 2:1.
C. HEF -EGF with a scale factor of root 3.1.
D. HEF -GEF with a scale factor of 3.1.

Answer 1

Option C

Explanation:

Two triangles are similar if they have at least 2 equal angles

Note that the HEF triangle has angles of 90 °, 60 ° and 30 °

Note that the EGF triangle has angles of 90 ° and 30 ° so the third angle must be 60 °

Then HEF and EGF are similar triangles.

By definition for similar triangles it is satisfied that if they have sides of length a, b, c and a ', b' c' then

`(a)/(a')=(b)/(b')=(c)/(c')=k`

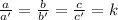
Where the constant k is known as "scale factor"

In this case

`(HF)/(EF)=(HE)/(EG)=(FE)/(GF)=k`

`k=(3)/(√(3))=(2√(3))/(2)=(√(3))/(1)=√(3)`

`(FE)/(GF)=(√(3))/(1)`

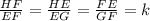
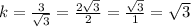
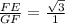
or

`√(3):1`

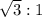
The answer is Option C

Answer 2

Option C.

Explanation:

we know that

If two triangles are similar, then the ratio of its corresponding sides is proportional and its corresponding angles are congruent

In this problem triangles HEF and EGE are similar

because

`EF/GF=HF/EF`

substitute the values

`(√(3))/(1)=(3)/(√(3))\\ \\3=3`

Is true

The sides are proportional

and

∠HFE=∠GFE

∠EHF=∠GEF

∠HEF=∠EGF

The angles are congruent

The scale factor is equal to

`EF/GF`

`(√(3))/(1)`