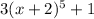Question

Which function results after applying the sequence of transformations to

f(x) = x^5?
• stretch vertically by 3
• translate up 1 unit
• translate left 2 units
​

Answer 1

f(x) = 3(x+2)⁵+1

Explanation:

Ap3x

Answer 2

The resulting function after the sequence of transformations is
`f(x)=3(x+2)^5+1`

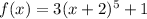
Explanation:

Given a function f(x)

• the function a*f(x) will be a vertical stretch of factor a, given a > 1
• the function f(x) + b will be the translated function vertically up b units
• the function f(x+c) will be horizontally translated function of the original by c units left

Remembering these points, we can apply the rules to
`x^5`.

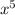• Strech vertically by 3 would be
  `3x^5`
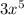
• translate up 1 unit would be
  `3x^(5)+1`
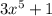
• translate left 2 units would make it
  `3(x+2)^5+1`

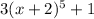
Hence the new function would be
`3(x+2)^5+1`