Answer:
(B)
Explanation:
Given: It is given that arc AD=130° and arc AB=arc CD=80°.
To find: The measure of ∠APD.
Solution: It is given that arc AD=130°⇒m∠AOD=130° (The measure of the central angel is equal to the intercepted arc)
Also, arc AB=arc CD=80°⇒m∠AOB=m∠DOC=80° (The measure of the central angel is equal to the intercepted arc)
We know that the sum of the central angles is equal to 360°, thus
m∠AOD+m∠AOB+m∠BOC+m∠COD=360°
⇒130°+80°+m∠BOC+80°=360°
⇒290°+m∠BOC=360°
⇒m∠BOC=360°-290°
⇒m∠BOC=70°
Now, since (The measure of the central angel is equal to the intercepted arc), therefore arcBC=70°.
Also, we know that Angle Formed by Two Secants is half of the DIFFERENCE of Intercepted Arcs, therefore
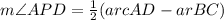
Substituting the values, we get

⇒
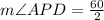
⇒
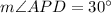
Thus, the measure of ∠APD is 30°.
Hence, option B is correct.