Question

Use factoring to solve the quadratic equation x2 − 2x − 3 = 12.

Answer 1

x = -3 and x = 5

Explanation:

x² − 2x − 3 = 12

x² − 2x − 3 - 12 = 0

x² − 2x − 15 = 0

Product = -15

sum = -2

Factors are: 3, -5

So the equation becomes;

x² − 2x − 15 = 0

x² + 3x - 5x − 15 = 0

(x² + 3x) - (5x + 15) = 0

x(x + 3) - 5(x + 3) = 0

(x + 3)(x - 5) = 0

∴ (x + 3) = 0

x = -3

or

(x - 5) = 0

x = 5

Answer 2

`x_1=5\\x_2=-3`

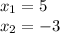
Explanation:

You have the following quadratic equation given in the problem:

`x^(2)-2x-3=12`

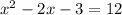
You must make the equation equal to zero, as following:

`x^(2)-2x-3-12=0`

Add like terms:

`x^(2)-2x-15=0`

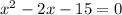
Now, to factor the equation, you must find two numbers whose sum is -2 and whose product is -15. Therefore, you have:

`(x-5)(x+3)=0\\x_1=5\\x_2=-3`