Answer:
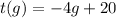
Step by step explanation:
Let g be the number of games James plays and t be the number of tokens James has.
We will write our function's formula in slope-intercept form of equation
 , where,
, where,
m = Slope of line,
b = y-intercept.
We have been given that initially James has 20 tokens. This means that before playing the games James has 20 tokens or at g equals 0 t equals 20. So our y-intercept will be 20.
We are also told that after playing the game 3 times, he has 8 tokens remaining.
Let us find the slope of line using points (3,8) and (0,20).
 , where,
, where,
m = Slope of line,
 = Difference between two y-coordinates,
= Difference between two y-coordinates,
 = Difference between x-coordinates of same two y-coordinates.
= Difference between x-coordinates of same two y-coordinates.
Upon substituting coordinates of our given points we will get,
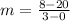
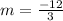
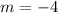
So the slope of our line will be -4.
We can see that number of remaining tokens are dependent on number games James plays, this mean that t is a function of g.
Upon substituting our values in slope-intercept form of equation we will get,
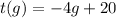 , where, t(g) represents number of tokens James has left after playing g games.
, where, t(g) represents number of tokens James has left after playing g games.
Therefore, our required function will be
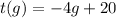 .
.