Question

The area of parallelogram WXYZ is approximately 45 square units. Trigonometric area formula: Area = 1/2absin(C). What is the approximate perimeter of the parallelogram? 5 units 15 units 30 units 40 units

Answer 1

C. 30 units. Just took the test and got it right

Answer 2

As, it is given that , Area of Parallelogram W X Y Z= 45 square units.

Area of Δ
`=(1)/(2)absinC`

As, diagonal of parallelogram divides it into two congruent triangles and congruent triangles have equal area.

So, Area (Δ W Y Z)= Area (Δ W X Y)

Area (Δ W X Z) =Area (Δ X Y Z)

Area (Δ WYZ)
`=(1)/(2)(WZ)*(ZY)sinZ`

`WZ*ZY Sin Z=22.5 *2\\\\ WZ* ZY*SinZ=45\\\\ Similarly, WX*XY*SinX=45\\\\ Similarly, WX*WZ*SinW=45\\\\ Similarly, XY*ZY*Sin Y=45`

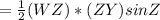
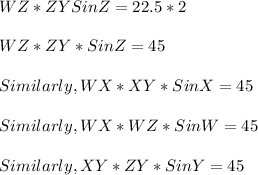
As, Opposite sides as well as Opposite angles of Parallelogram are equal.

Adding all the equation written above,

2(WZ*ZY*Sin Z )+ 2(WX*WZ*SinW)=180

2 * WZ *ZY(Sin Z +Sin W)=180

WZ *ZY(Sin Z +Sin W)=90

Consider the case when , SinZ=SinW=1, when the parallelogram turns into rectangle.

Area of Parallelogram which is rectangle =45 square units

L*B=45

Perimeter of Parallelogram = 2 * (L +B)

`P=2 L +2* (45)/(L)\\\\ P =2 L + (90)/(L)`

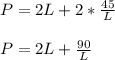
Differentiating Once w.r.t L

`P'=2-(90)/(L^2)`

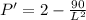
For maxima or minima

L²=45

L= 6.70 units

Gives, B= 6.72 units

Perimeter = 2 × (L +B)

= 2 × (6.70 +6.72)

=2 × 13.42

= 26.84

As, area of parallelogram is smaller than area of rectangle having same dimension.

So, Approximate perimeter of Parallelogram = 30 units.

Option C: 30 units