Question

Sarah is a computer engineer and manager and works for a software company. She receives a

fixed annual increase in the number of projects she handles each year. She worked on 129

projects in the fourth year of her employment and 207 projects in her tenth year.

(a) How many projects did Sarah work on in her first year?

(b) If a project pays her $500 on average,

I. How much did she earn in her twelfth year?

II. How much did she earn in total in her first 12 years with the company

Answer 1

a) Number of projects in the first year = 90

b) Earnings in the twelfth year = $116500

Total money earned in 12 years = $969000

Explanation:

Given that:

Number of projects done in fourth year = 129

Number of projects done in tenth year = 207

There is a fixed increase every year.

a) To find:

Number of projects done in the first year.

This problem is nothing but a case of arithmetic progression.

Let the first term i.e. number of projects done in first year =
`a`

Given that:

`a_4=129\\a_(10)=207`

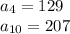
Formula for
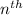`n^(th)` term of an Arithmetic Progression is given as:

`a_n=a+(n-1)d`

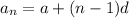
Where
`d` will represent the number of projects increased every year.

and
`n` is the year number.

`a_4=129=a+(4-1)d \\\Rightarrow 129=a+3d .....(1)\\a_(10)=207=a+(10-1)d \\\Rightarrow 207=a+9d .....(2)`

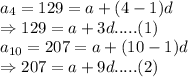
Subtracting (2) from (1):

`78 = 6d\\\Rightarrow d =13`

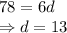
By equation (1):

`129 =a+3* 13\\\Rightarrow a =129-39\\\Rightarrow a =90`

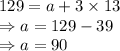
Number of projects in the first year = 90

b)

Number of projects in the twelfth year =

`a_(12) = a+11d\\\Rightarrow a_(12) = 90+11* 13 =233`

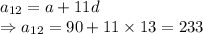
Each project pays $500

Earnings in the twelfth year = 233
`*` 500 = $116500

Sum of an AP is given as:

`S_n=(n)/(2)(2a+(n-1)d)\\\Rightarrow S_(12)=(12)/(2)(2* 90+(12-1)* 13)\\\Rightarrow S_(12)=6* 323\\\Rightarrow S_(12)=1938`

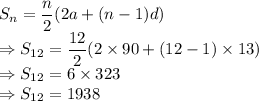
It gives us the total number of projects done in 12 years = 1938

Total money earned in 12 years = 500
`*` 1938 = $969000