Question

Drum lessons at the youth club cost $8 each for members. There is a membership fee of $24. For non membership, each drum lessons cost $12. For what number of lessons is the cost the same for a member and non member

Answer 1

6

Explanation:

Given that:

One time membership fee = $24

Cost for each drum lesson at the youth club for members = $8

For non-members,

Cost for each drum lesson = $12

To find:

Number of drum lessons such that the cost for a member and a non-members is same.

Solution:

Let the number of drum lessons =
`x`

Cost for drum lessons for the members = Number of drum lessons
`*` Cost for one drum lesson

Cost for drum lessons for the members = $
`8x`

Total cost for members = $24 + $
`8x`

Total cost for the drum lessons for the non-members = $12
`x`

As per question statement, both the expressions are equal:

`24+8x = 12x\\\Rightarrow 12x-8x=24\\\Rightarrow 4x =24\\\Rightarrow x = 6`

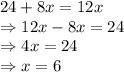
For 6 number of lessons, cost for both will be same.