Answer:
![\displaystyle \large{f(4)=22}\\\\\displaystyle \large{f^(-1)(x)=\sqrt[3]{4x-24}}\\\\\displaystyle \large{f^(-1)(8) = 2}](https://img.qammunity.org/2023/formulas/mathematics/high-school/2up5k5p3xgkflbipq7uqr14euxg603nay8.png)
Explanation:
In this problem, we are given the linear function:
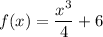
( a ) Find f(4)
Simply substitute x = 4 in the function f(x).
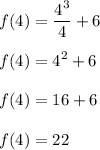
( b ) Find the inverse
To find
 , solve for x-term then swap x-term and y-term.
, solve for x-term then swap x-term and y-term.
![\displaystyle \large{4f(x)=x^3+24}\\\\\displaystyle \large{4f(x)-24=x^3}\\\\\displaystyle \large{\sqrt[3]{4f(x)-24}=\sqrt[3]{x^3}}\\\\\displaystyle \large{x=\sqrt[3]{4f(x)-24}}](https://img.qammunity.org/2023/formulas/mathematics/high-school/kxworqqihsqq42cgzilvha4gcoiaods8rq.png)
Swap f(x) and x.
![\displaystyle \large{f^(-1)(x)=\sqrt[3]{4x-24}}](https://img.qammunity.org/2023/formulas/mathematics/high-school/wdb35uuy8kfmr5q4qfdv8u6oygx6gw4l7e.png)
( c ) Find inverse f(8)
Substitute x = 8 in inverse function.
![\displaystyle \large{f^(-1)(8) = \sqrt[3]{4(8)-24}}\\\\\displaystyle \large{f^(-1)(8) = \sqrt[3]{32-24}}\\\\\displaystyle \large{f^(-1)(8) = \sqrt[3]{8}}\\\\\displaystyle \large{f^(-1)(8) = 2}](https://img.qammunity.org/2023/formulas/mathematics/high-school/negxqk8cv3dhczsukorq5fvavjeyqho9pl.png)
Please let me know if you have any doubts!