Question

:pppppppppppp here it is again

Answer 1

Below

Explanation:

Given Data:

• The sum of two numbers is less than 2
• If we subtract the second number from the first , the difference is greater than 1

Q) What are the two numbers?

Lets suppose the first number to be x and the second number to be y

The first point states sum of two number which is x + y is less than 2 so our first expression becomes,

`x+y<2`

The second point states that the if we subtract the second number from the first number which states x - y the difference is greater than 1 so our second expression becomes,

`x-y<1`

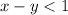
1) The riddle can be represented by a system of inequalities. Write an inequality for each statement.

The inequality for the first statement is

`x+y<2`

The inequality for the second statement is

`x-y>1\\`

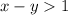
I assume the second part to the question would be solve for x and y xD

so here goes

2)

If we just simply add both the equations we get the following result:

`x+x+y-y=2+1`

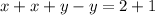
Just suppose there is an equal to sign instead of less than or greater than

`x+x+y-y=2+1\\2x+0=3\\x=3/2\\x=1.5\\`

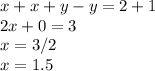
now put the value of x in any one of the two equations I choose equation 1

`x+y=2\\1.5+y=2\\y=2-1.5\\y=0.5`

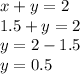
I attached an image so you can understand it better :)

Answer 2

i think just any negative numbers

Explanation:

like for example -10 and -2. their sum is -12 and when subtracted is -8