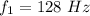Question

One of the harmonics of a column of air in a tube that is open at both ends has a frequency of 448 Hz, and the next higher harmonic has a frequency of 576 Hz. What is the fundamental frequency of the air column in this tube?

Answer 1

The fundamental frequency is
`f_1 =128 \ Hz`

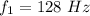
Step-by-step explanation:

From the question we are told that

The frequency of one harmonics is
`f_x= 448 \ Hz`

The next higher harmonic is
`f_z = 576 \ Hz`

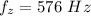
Generally the frequency of an air column open at both ends is mathematically represented as

`f_n = (nv )/( 2 L )`

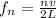
Here n is the order of the harmonics (frequency)

v is the velocity of the sound

L is the length of the column

So for one harmonics we have that

`f_k = (n v )/(2L)`

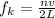
Then for the next higher harmonics

`f_x = (n+1 ) v)/(2 L )`

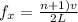
Generally the difference between these frequencies is mathematically represented as

`f_z- f_x = ((n+1 )v)/( 2L) - ((n )v)/( 2L)`

=>
`576 - 448 = (vn + v - nv )/(2L)`

=>
`( v )/(2L) = 128`

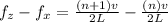
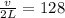
Generally for fundamental frequency n = 1

So

`f_1 = n * (v)/(2L)`

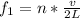
So

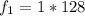
`f_1 =1 * 128`

=>
`f_1 =128 \ Hz`