Answer:
The equivalent will be:
![\frac{\sqrt[7]{x^2}}{\sqrt[5]{y^3}}=\left(\:x^{(2)/(7)}\right)\left(y^{-(3)/(5)}\right)](https://img.qammunity.org/2021/formulas/mathematics/high-school/6a87rl0t1wrr8uzxy7u4bwg6ccvi8vq5ki.png)
Therefore, option 'a' is true.
Explanation:
Given the expression
![\frac{\sqrt[7]{x^2}}{\sqrt[5]{y^3}}](https://img.qammunity.org/2021/formulas/mathematics/high-school/dj72ba9ccqglkgtb0c8t5enkbdwyxi94yc.png)
Let us solve the expression step by step to get the equivalent
![\frac{\sqrt[7]{x^2}}{\sqrt[5]{y^3}}](https://img.qammunity.org/2021/formulas/mathematics/high-school/dj72ba9ccqglkgtb0c8t5enkbdwyxi94yc.png)
as
![\sqrt[7]{x^2}=\left(x^2\right)^{(1)/(7)}](https://img.qammunity.org/2021/formulas/mathematics/high-school/kr5000nixmh49rz95n1js75978ip5yje0x.png) ∵
∵
![\mathrm{Apply\:radical\:rule}:\quad \sqrt[n]{a}=a^{(1)/(n)}](https://img.qammunity.org/2021/formulas/mathematics/high-school/u9jioc8r7jc4x6939q2vaxxxup1pf8r1ej.png)
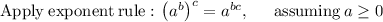
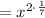
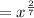
also
![\sqrt[5]{y^3}=\left(y^3\right)^{(1)/(5)}](https://img.qammunity.org/2021/formulas/mathematics/high-school/99x2t5lk2wtcfxdiilto5l6ap4al4ciy7e.png) ∵
∵
![\mathrm{Apply\:radical\:rule}:\quad \sqrt[n]{a}=a^{(1)/(n)}](https://img.qammunity.org/2021/formulas/mathematics/high-school/u9jioc8r7jc4x6939q2vaxxxup1pf8r1ej.png)
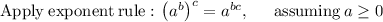
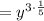

so the expression becomes
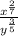
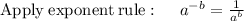
 ∵
∵
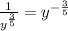
Thus, the equivalent will be:
![\frac{\sqrt[7]{x^2}}{\sqrt[5]{y^3}}=\left(\:x^{(2)/(7)}\right)\left(y^{-(3)/(5)}\right)](https://img.qammunity.org/2021/formulas/mathematics/high-school/6a87rl0t1wrr8uzxy7u4bwg6ccvi8vq5ki.png)
Therefore, option 'a' is true.