Answer:
a
Explanation:
given a quadratic equation in standard form
ax² + bx + c = 0 ( a ≠ 0 )
then the discriminant
Δ = b² - 4ac
• if b² - 4ac > 0 then 2 real solutions
• if b² - 4ac = 0 then 2 real and equal solutions
• if b² - 4ac < 0 then no real solutions
given
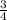 x² - 3x = - 4 ( add 4 to both sides )
x² - 3x = - 4 ( add 4 to both sides )
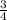 x² - 3x + 4 = 0 ← in standard form
x² - 3x + 4 = 0 ← in standard form
with a =
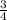 b = - 3 , c = 4
b = - 3 , c = 4
then
b² - 4ac = (- 3)² - ( 4 ×
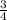 × 4) = 9 - 12 = - 3
× 4) = 9 - 12 = - 3
since b² - 4ac < 0 then equation has no real solutions