Answer:
The range is:
{-4, 1, 2, 5, 8}
Explanation:
Given the function
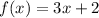
- We know that the domain of a function is the set of input or argument values for which the function is real and defined.
As the domain interval -2 ≤ x ≤ 2
i.e. the values in the domain = {-2, -1, 0, 1, 2}
- We also know that the range of a function is the set of values of the dependent variable for which a function is defined.
As the domain interval -2 ≤ x ≤ 2
Putting all the x-values in the domain interval in the function
so
putting x=-2 in the function
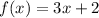
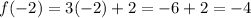
putting x=-1 in the function
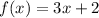
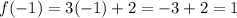
putting x=0 in the function
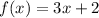
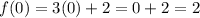
putting x=1 in the function
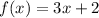
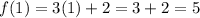
putting x=2 in the function
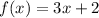

Thus, when we put the domain values, the corresponding range values are:
x y
-2 -4
-1 1
0 2
1 5
2 8
Therefore, the range is:
{-4, 1, 2, 5, 8}