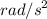The question is not complete. The complete question is :
During a certain period of time, the angular position of a rotating object is given by
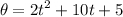 , where θ is in radians and t is in seconds. Determine the angular position, angular speed, and angular acceleration of the door (a) at t = 0.00 seconds, (b) at t = 3.00 seconds.
, where θ is in radians and t is in seconds. Determine the angular position, angular speed, and angular acceleration of the door (a) at t = 0.00 seconds, (b) at t = 3.00 seconds.
Solution :
Given :
Displacement or angular position of the object,
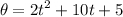
∴ Angular speed is
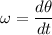
ω = 10 + 4t
And angular acceleration is
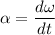
α = 4
a). At time, t = 0.00 seconds :
Angular displacement is
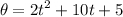
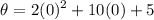
= 5 rad
Angular speed is ω = 10 + 4t
ω = 10 + 4(0)
= 10 rad/s
Angular acceleration is α = 4
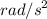
b). At time, t = 3.00 seconds :
Angular displacement is
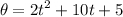

= 53 rad
Angular speed is ω = 10 + 4t
ω = 10 + 4(3)
= 22 rad/s
Angular acceleration is α = 4