Question

Here is a probability scale:

0
0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9

There are 3 white counters and 1 black counter in a bag.
I take one of the counters at random.
Put a cross on the probability scale to show the probability
that I have chosen the black counter.

Answer 1

0.25

Explanation:

3 white counters and 1 black counter,

3+1 = 4

the chances of you choosing the black counter is 1/4

1/4 can also be written as 0.25.

Answer 2

The probability of choosing a black counter is 0.25.

Also the picture is attached for the mark on scale.

Explanation:

Probability is the likeliness of occurrence of an event.

Given

There are three white and 1 black counter in the bag which means our sample space contains 3+1 = 4 elements

n(S) = 4

Let B be the event that the drawn out counter is black. As there is only one black counter

n(B) = 1

The probability of choosing a black counter is:

`P(B) = (n(B))/(n(S))\\P(B) = (1)/(4) = 0.25`

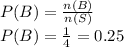
Hence,

The probability of choosing a black counter is 0.25.

Also the picture is attached for the mark on scale.