Question

Question 15 (1 point)

The surface area of a sphere varies directly with the square of its radius. A soap bubble with a
0.75 in. radius has a surface area of approximately 7.07 square inches. Find the value of k, and
then find the radius of a seventeenth-century cannonball that has a surface area of 113.1
square inches.

Answer 1

• The value of k is 12.57
• Radius of a seventeenth-century cannonball is 2.999 inches which can be rounded off to 3

Explanation:

Let s be the surface area and r be the radius

Then according to given statement

s∝r²

Removing the proportionality symbol introduces k, the constant of proportionality

`s = kr^2`

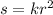
Now

A soap bubble with a 0.75 in. radius has a surface area of approximately 7.07 square inches.

Putting in the equation

`7.07 = k (0.75)^2\\7.07 = k * 0.5625\\k = (7.07)/(0.5625)\\k = 12.5688..\\k = 12.57`

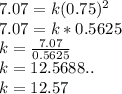
The euqation beomes

`s = 12.57r^2`

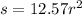
Putting s = 113.1 in the equation

`113.1 = 12.57r^2\\r^2 = (113.1)/(12.57)\\r^2 = 8.99761...\\√(r^2) = √(8.9976..)\\r = 2.9996..`

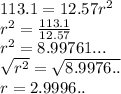
Hence,

• The value of k is 12.57
• Radius of a seventeenth-century cannonball is 2.999 inches which can be rounded off to 3