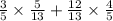Question

If sinA 3/5 and cos B 5/13 , then find the value of sin (A+B)​

Answer 1

`(63)/(65)`

Explanation:

We have to find the value of sinB & cosA . So to find the value of sinB , let's use the identity

`{ \sin }^(2) b + { \cos }^(2) b = 1`

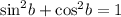
By using the identity above gives

`{ \sin}^(2) b + ( { (5 )/(13) })^(2) = 1`

`= > { \sin}^(2) b = 1 - (25)/(169) = (144)/(169)`

`= > \sin(b) = \sqrt{ (144)/(169) } = (12)/(13)`

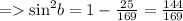
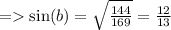
Now to find the value of cosA , we'll use the same identity.

`{( (3)/(5) })^(2) + { \cos}^(2) a = 1`

`= > { \cos }^(2) a = 1 - (9)/(25) = (16)/(25)`

`= > \cos(a) = \sqrt{ (16)/(25) } = (4)/(5)`

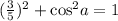
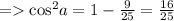
Now we know that

`\sin(a + b)=\sin(a)\cos(b)+\sin(b) \cos(a)`

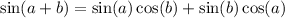
So value of sin(a+b) =

`(3)/(5) * (5)/(13) + (12)/(13) * (4)/(5)`

`= (3)/(13) + (48)/(65)`

`= (63)/(65)`