Answer:
- 26 m/s
- 53 1/3 m
Explanation:
The speed will be the initial speed plus the integral of acceleration:
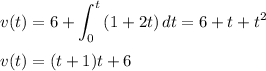
The position will be the integral of speed:
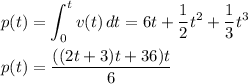
__
1. The velocity at t=4 is v(4) = (4+1)4 +6 = 26 m/s
2. The position at t=4 = ((2·4 +3)4 +36)4/6 = (44 +36)(2/3) = 160/3 = 53 1/3 m
At t=4, the particle will be 53 1/3 m from O and will be moving at a speed of 26 m/s.