Question

What is the value of y if x is 8 in the linear equation graphed?

A) 4
B) 5
C) 7
D) 13

Answer 1

When x = 8, our y-value is equal to 13.

Explanation:

We are given a graph that shows a linear relationship between our variables x and y.

In math, the slope-intercept form of an equation is written as:

`\bullet \ y=mx+b, \text{where m is our slope and b is our y-intercept.}`

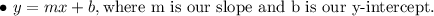
We also can note that there is another way to state a function:
`f(x)`. This replaces the y in the slope-intercept form and they can be used interchangeably.

`\bullet \ f(x) = mx + b`

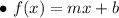
So, when we look at our graph, we can see that we have x-values on the bottom horizontal axis and y-values on the left vertical axis.

When we check this, we see that our x-values range from 0 to 9 and our y-values range from -2 to 14.

Therefore, we can see that since x is supposed to be equal to 8, we are going to draw a vertical line from x = 8 and see what point this intersects.

We see that the line would intersect the rightmost point and it is located between y = 12 and y = 14.

Therefore, we can just take the median of these two values and find our y value.

`\displaystyle 12 + 14 = 26\\\\(26)/(2) = 13`

Therefore, y is equal to 13 and our answer is Choice D.