Question

Explain how to determine if the two expressions are equivalent using x=6 and x=10

8x+40. 8(x+5)

Answer 1

Explanation:

if they're equivalent, when you plug in either 10 or 6 for x, they should both equal the same.

x=10, 8(10)+40=120.... 8(10+5)=120. so they equal. also test 6

8(6)+40=88. 8(6+5)=88

they are equivalent since they both equal each other, no matter what you plug in. reason being that the 8(x+5) is the factorized version of 8x+40

Answer 2

By putting both values of x in the expression, it can be concluded that both expressions are equivalent.

Explanation:

The expressions can be evaluated to be equivalent by putting the values given

The expressions are:

8x+40 and 8(x+5)

Putting x = 6 in both

`8x+40\ and\ 8(x+5)\\8(6)+40\ \ ; \ 8(5+6)\\48+40\ \ \ \ ; \ \ 8(11)\\88\ \ \ \ \ \ \ \ \ \ \ ; \ \ 88`

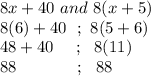
The values of both expressions are same on x=6

Now putting x = 10

`8x+40\ and\ 8(x+5)\\8(10)+40\ \ ; \ 8(10+6)\\80+40\ \ \ \ ; \ \ 8(16)\\120\ \ \ \ \ \ \ \ \ ; \ \ 120`

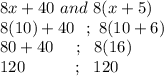
The values of both expressions are same on x = 10

Hence,

By putting both values of x in the expression, it can be concluded that both expressions are equivalent.