Question

Which option correctly shows how this formula can be rearranged to isolate x^2?

Options in picture.

Answer 1

A

Explanation:

We have:

`\displaystyle m=(x_1-x_2)/(y_1-y_2)`

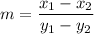
And we want to isolate x₂.

So, let’s first remove the denominator by multiplying both sides by it:

`\displaystyle m(y_1-y_2)=(x_1-x_2)/(y_1-y_2)(y_1-y_2)`

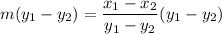
The right side will cancel. This will leave:

`\displaystyle m(y_1-y_2)=x_1-x_2`

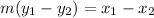
Now, we can subtract x₁ from both sides. So:

`\displaystyle m(y_1-y_2)-x_1=-x_2`

Finally, we will multiply both sides by -1. So:

`\displaystyle x_2=-m(y_1-y_2)+x_1`

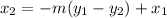
Hence, our answer is A.