Question

Solve the following pair of equations simultaneously.

See image for question
Answer question (III)

Answer 1

No solutions.

General Formulas and Concepts:

Pre-Algebra

• Order of Operations: BPEMDAS
• Equality Properties

Algebra I

• Solving systems of equations using substitution/elimination
• Solving systems of equations by graphing
• Expanding
• Finding roots of a quadratic
• Standard Form: ax² + bx + c = 0
• Quadratic Formula:
  `x=(-b\pm√(b^2-4ac) )/(2a)`

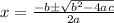
Explanation:

Step 1: Define systems

2x - y = 9

4x² + 3y² - 2x + y = 16

Step 2: Rewrite systems

2x - y = 9

1. Subtract 2x on both sides: -y = 9 - 2x
2. Divide -1 on both sides: y = 2x - 9

Step 3: Redefine systems

y = 2x - 9

4x² + 3y² - 2x + y = 16

Step 4: Solve for x

Substitution

1. Substitute in y: 4x² + 3(2x - 9)² - 2x + (2x - 9) = 16
2. Expand: 4x² + 3(4x² - 36x + 81) - 2x + (2x - 9) = 16
3. Distribute 3: 4x² + 12x² - 108x + 243 - 2x + 2x - 9 = 16
4. Combine like terms: 16x² - 108x + 234 = 16
5. Factor GCF: 2(8x² - 54x + 117) = 16
6. Divide 2 on both sides: 8x² - 54x + 117 = 8
7. Subtract 8 on both sides: 8x² - 54x + 109 = 0
8. Define variables: a = 8, b = -54, c = 109
9. Resubstitute:
   `x=(54\pm√((-54)^2-4(8)(109)) )/(2(8))`
10. Exponents:
    `x=(54\pm√(2916-4(8)(109)) )/(2(8))`
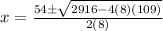
11. Multiply:
    `x=(54\pm√(2916-3488) )/(16)`
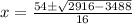
12. Subtract:
    `x=(54\pm√(-572) )/(16)`

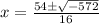
Here we see that we start to delve into imaginary roots. Since on a real number plane, we do not have imaginary roots, there would be no solution to the systems of equations.

Step 5: Graph systems

We can verify our results.