Question

A freshly caught catfish is placed on a spring scale, and it oscillates up and down with a period of 0.19 s. If the spring constant of the scale is 2330 N/m, what is the mass of the catfish?

Answer 1

The mass of the catfish is 2.13 kg

Step-by-step explanation:

Period of oscillation, T = 0.19 s

spring constant, k = 2330 N/m

The period of oscillation of the spring is given by;

`T = 2\pi \sqrt{(m)/(k) }\\\\(T)/(2\pi) = \sqrt{(m)/(k) }\\\\(T^2)/(4\pi^2) = (m)/(k)\\\\m = (kT^2)/(4\pi^2)`

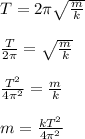
where;

m is mass of the catfish

substitute the given values and solve for m;

`m = (kT^2)/(4\pi^2) \\\\m = ((2330)(0.19)^2)/(4\pi^2) \\\\m = 2.13 \ kg`

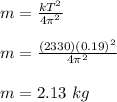
Therefore, the mass of the catfish is 2.13 kg