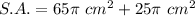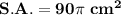Answer:
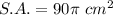
Explanation:
Surface Area of a Cone
Given a cone of base area B and slant height L, the surface area can be calculated with the formula:
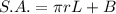
The cone presented in the image has a circular base of radius r=5 cm, thus its base area is:
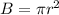
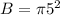
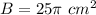
The slant height can be calculated as the hypotenuse of a right triangle with legs 5 cm and 12 cm by using Pythatgora's Theorem:
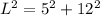
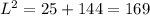
Taking square roots
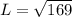
L = 13 cm
The surface area is:

Operating: