Answer:
- The length of VW = 36 units.
Explanation:
We know that a rhombus is a quadrilateral with four sides. All four sides have the same length.
From the diagram, it is clear that
As all the four sides of the rhombus have equal length.
So we can observe
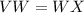
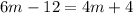
Add 12 to both sides
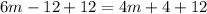
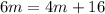
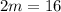
Divide both sides by 2

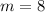
so
The length of VW= 6m-12
= 6(8)-12 ∵
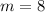
= 48-12
= 36
Thereofore, the length of VW = 36 units.