Answer:
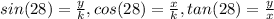
Explanation:
For this problem, k is the hypotenuse, x is the side adjacent to 28, and y is the side opposite 28.
I would remember SOH CAH TOA.
S - Sine
O - Opposite
H - Hypotenuse
For this, it shows that the sine of the angle is opposite over hypotenuse. Therefore, y/k.
C - Cosine
A - Adjacent
H - Hypotenuse
For this, it shows that the cosine of the angle is adjacent over hypotenuse. Therefore, x/k
T - Tangent
O - Opposite
A - Adjacent
For this, it shows that the tangent of the angle is opposite over adjacent. Therefore, y/x